HDH Desalination | Lienhard Research Group

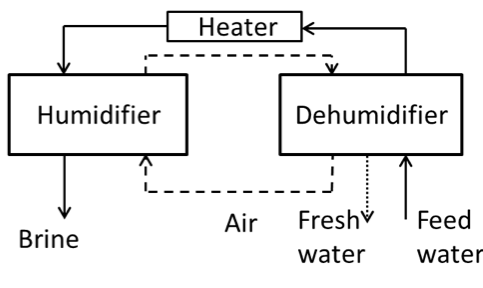
Humidification-dehumidification (HDH) is a thermal desalination technology that imitates nature’s rain cycle. Its basic components are a humidifier, a dehumidifier, and a heater. In the humidifier, air is humidified through direct contact with salty water. In the dehumidifier, hot moist air is put in indirect contact with cold salty water. This causes water vapor to condense, which produces a fresh water stream.

A humidification-dehumidification system can be configured in multiple ways by varying which stream gets heated, and whether streams are circulated in a closed loop or leave the system after one pass. Narayan et al. [14] have listed the merits of the different HDH configurations.

Schematic diagram of a typical HDH cycle

Figure 1. Schematic diagram of a typical humidification dehumidification desalination cycle

The main energy input to an HDH system is thermal energy to the heater. If the system produces purified water at a rate of $\dot{m}_p$ (kg/s) and the heat input is $\dot{Q}_h$ (W), we may describe the energy efficiency in a first law sense using the Gained-Output-Ratio, or GOR:

(1) $\begin{equation*} \text{GOR} = \frac{h_{\text{fg}}\dot{m}_p}{\dot{Q}_h} \end{equation*}$

where $h_{\text{fg}}$ is the latent heat of vaporization (J/kg).

If the system simply vaporized water and condensed it without any energy recovery, GOR would be 1 at most (heat losses and net temperature rise of discharged fluids would lower efficiency further). In a well-designed system, the heat of condensation is recovered by preheating the incoming feed in the dehumidifier (through techniques of balancing and multi-staging as described below). With effective energy recovery, GOR values several times higher may be obtained. Electrical energy is also needed to drive pumps and blowers.

Effectiveness of heat and mass exchangers:
When studying a heat and mass exchanger it is important to define an exchanger effectiveness to determine how close the exchanger is to maximum (infinite area) performance. For example, an effectiveness of 50% means that having an effectively infinite surface area would double the amount of energy transferred.

In addition, the exchanger efficiency can be used to model a more complex HDH system without the need to develop a heat and mass transfer model. The performance of the dehumidifier and the humidifier can be determined by a value of their effectiveness.

The energy effectiveness of heat and mass exchangers was defined by Narayan et al. [1] and was generalized by Chehayeb et al. [2] to include a working definition of the effectiveness of humidifiers where the pinch occurs at an intermediate location:

(2) $\begin{equation*} \varepsilon = \frac{\Delta \dot{H}}{\Delta \dot{H}_{\rm max}} = \frac{\Delta \dot{H}}{\Delta\dot{H}_{\rm pinch = 0}} \end{equation*}$

where, in a dehumidifier, the pinch occurs at one of the outlets of the exchanger:

(3) $\begin{equation*} \Delta \dot{H}_{\rm pinch = 0, deh} = \text{min}\,\left(\Delta \dot{H}_{\rm max, water}, \Delta \dot{H}_{\rm max, air}\right) \end{equation*}$

In contrast, for the humidifier, the pinch occurs at an intermediate location:

(4) $\begin{equation*} \Delta \dot{H}_{\rm pinch = 0, hum} = \Delta \dot{H}+ \dot{m}_{\text{dry air}}\Psi_{\rm hum} \end{equation*}$

In this expression, $\Psi$ is the enthalpy pinch in the humidifier as defined by Narayan et al. [7].

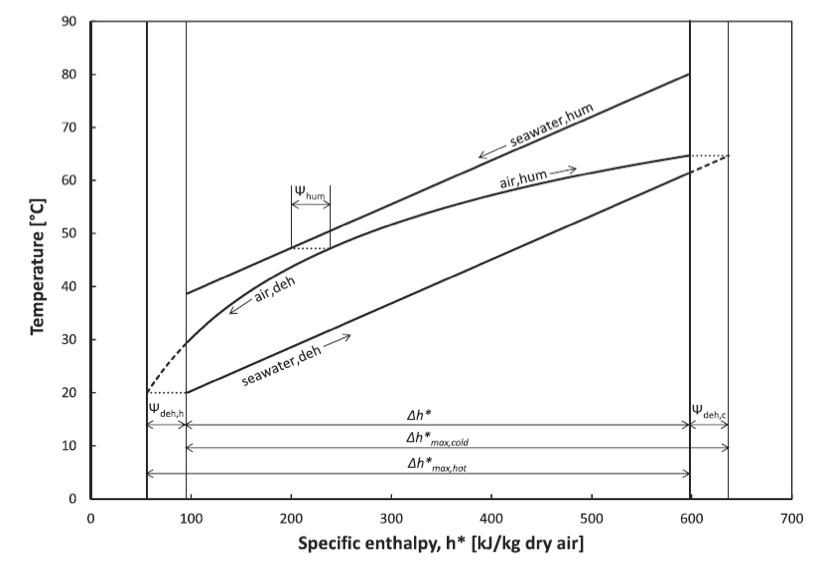
Figure 2. Temperature-enthalpy diagram showing the enthalpy pinch and the maximum changes in the enthalpy rate.

Thermodynamic balancing of humidification-dehumidification:
Given that HDH is a combination of heat and mass exchangers, its performance is strongly dependent on the mass flow rates of the interacting streams, namely air and water. For this reason, it is important to define the water-to-air mass flow rate ratio, MR:

(5) $\begin{equation*} \text{MR} = \frac{\dot{m}_{\rm water}}{\dot{m}_{\rm dry air}} \end{equation*}$

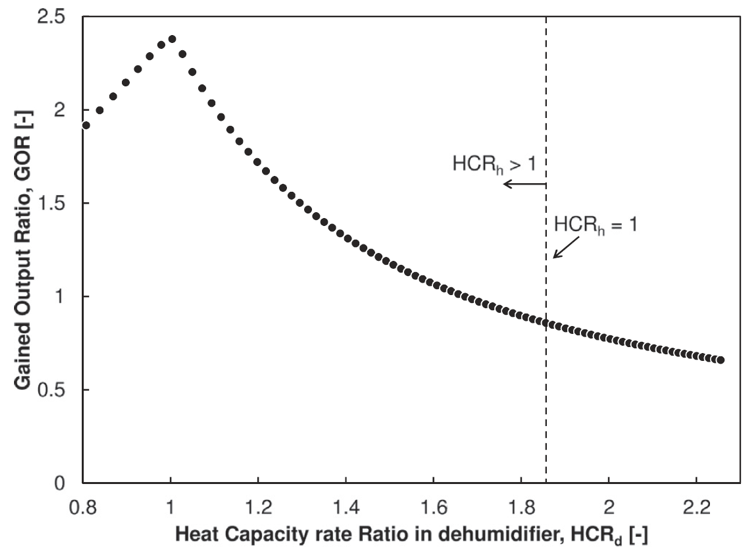
Mistry et al. [3] have shown that the highest energy efficiency (GOR) is achieved when entropy generation is minimized. This occurs at one value of the mass flow rate ratio.

Figure 3. GOR is maximized and specific entropy generation is minimized at the same mass flow rate ratio

In simultaneous heat and mass exchangers, the enthalpy rates of the air stream vary not only with temperature but also with humidity. Consequently, a simple mass flow rate ratio is insufficient to describe the energy transfers between streams. Instead, Narayan et al. [4] defined the modified heat capacity rate ratio, or HCR, in terms of enthalpy flow rates as:

(6) $\begin{equation*} \text{HCR} = \frac{\Delta \dot{H}_{\rm max, cold}}{\Delta \dot{H}_{\rm max, hot}} \end{equation*}$

Further, Narayan et al. [4] and Chehayeb et al. [2] showed that the optimal mass flow rate ratio also results in HCR = 1 in the dehumidifier, which can be used as a condition for maximizing GOR.

Figure 4. Variation of GOR with HCR_d

These trends can best be explained by looking at the distribution of entropy generation along the length of the components, as was done by Thiel et al. [5].

Varying the mass flow rate ratio through multi-staging:
The benefits of having a balanced system can be extended by operating the system under multiple mass flow rate ratios, or multiple stages. These benefits were shown by McGovern et al. [6], who modeled single-stage and two-stage systems by using a temperature pinch, by Narayan et al. [7], who used an enthalpy pinch to study the performance of single-stage, two-stage, and infinitely-staged systems, and by Chehayeb et al. [8], who extended the analysis to systems having up to 6 stages.

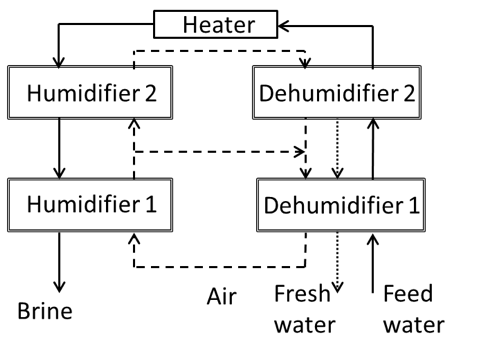
Figure 5. Schematic diagram representing a water-heated, closed-air, open-water HDH system with a single air extraction.

Although fixed-effectiveness (or fixed-pinch) modeling is useful in understanding the benefits of multi-staging, these benefits can only be isolated and quantified when a fixed area is used. Chehayeb et al. [2] modeled a system of fixed-area and provided guidelines for the proper operation of single-stage and two-stage systems, including the proper direction of extraction. In addition, the effect of a single extraction on a system of fixed size was experimentally studied by Narayan et al. [9].

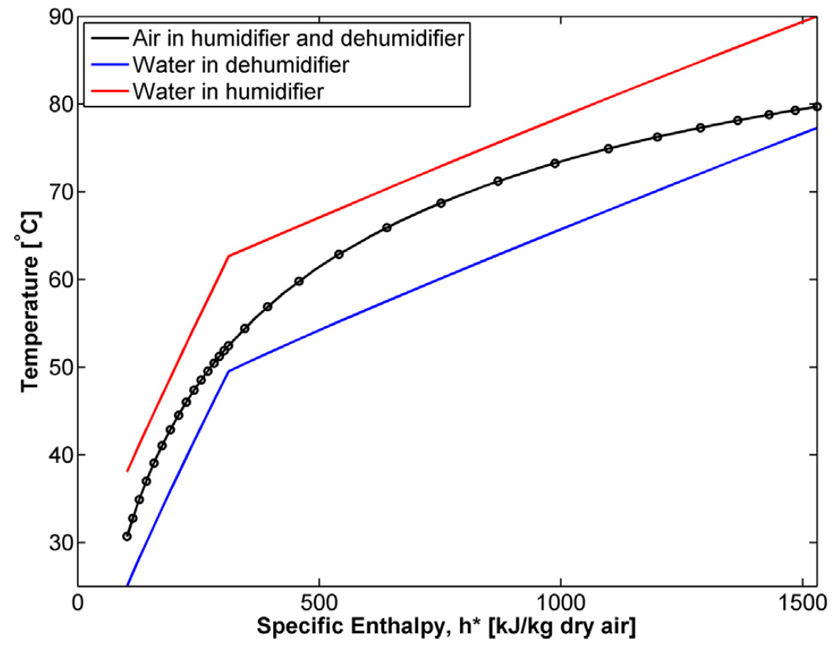
Figure 6. Temperature-enthalpy diagram of a two-stage HDH system. A better distribution of the driving forces has led to a GOR increase of 58% compared to a single-stage system of the same size.

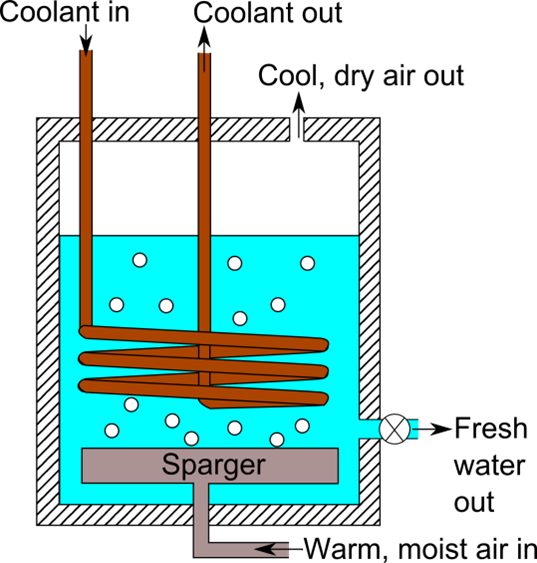
Bubble column dehumidifiers:
Narayan et al. [10] proposed the use of bubble columns as dehumidifiers in the HDH system to reduce cost. In a bubble column dehumidifier, condensation occurs at the surface of air bubbles rather than an expensive metal surface. Tow and Lienhard [11] developed a model for a bubble column dehumidifier and validated it with experimental data. They also developed a correlation for the heat transfer coefficient of a cylinder submerged in a shallow bubble column [12]. Turbulence created by rising bubbles leads to high heat transfer coefficients (>5000 W/m²K) at the surface of the cooling element, reducing the material cost associated with condensation.

Figure 7. Schematic drawing of a bubble column dehumidifier.

For further reading:

Lienhard has prepared a book chapter that introduces humidification-dehumidification desalination [13].

References:

[1] G.P. Narayan, K.H. Mistry, M.H. Sharqawy, S.M. Zubair, J.H. Lienhard V, “Energy Effectiveness of Simultaneous Heat and Mass Exchange Devices,” Frontiers in Heat and Mass Transfer, 1(2):1-13, Aug. 2010. (doi link) (pdf)

[2] K.M. Chehayeb, G.P. Narayan, S.M. Zubair, and J.H. Lienhard V, “Thermodynamic balancing of a fixed-size two-stage humidification dehumidification desalination system,” Desalination, 369:125-139, 3 August 2015; online 16 May 2015. (doi link) (preprint)

[3] K.H. Mistry, J.H. Lienhard V, and S.M. Zubair, “Effect of Entropy Generation on the Performance of Humidification-Dehumidification Desalination Cycles,” International Journal of Thermal Sciences, 49(9):1837-1847, Sept. 2010. (doi link) (pdf)

[4] G.P. Narayan, M.H. Sharqawy, J.H. Lienhard V, and S.M. Zubair, “Thermodynamic analysis of humidification-dehumidification desalination cycles,” Desalination and Water Treatment, 16:339-353, April 2010. (pdf)

[5] G.P. Thiel, R.K. McGovern, S.M. Zubair, and J.H. Lienhard V, “Thermodynamic equipartition for increased second law efficiency,” Applied Energy, 118C:292-299, April 2014. (doi link) (preprint)

[6] R.K. McGovern, G.P. Thiel, G.P. Narayan, S.M. Zubair, and J.H. Lienhard V, “Performance Limits of Single and Dual Stage Humidification Dehumidification Desalination Systems,” Applied Energy, 102:1081-1090, Feb. 2013. (doi link) (preprint)

[7] G.P. Narayan, K.M. Chehayeb, R.K. McGovern, G.P. Thiel, S.M. Zubair, and J.H. Lienhard V, “Thermodynamic balancing of the humidification dehumidification desalination system by mass extraction and injection,” International Journal of Heat and Mass Transfer, 57(2):756-770, Feb. 2013 (doi link) (preprint)

[8] K.M. Chehayeb, G.P. Narayan, S.M. Zubair, and J.H. Lienhard V, “Use of multiple extractions and injections to thermodynamically balance the humidification dehumidification desalination system,” Intl. J. Heat Mass Transfer, 68:422–434, Jan. 2014. (doi link) (preprint)

[9] G.P. Narayan, M.S. St. John, S.M. Zubair, and J.H. Lienhard V, “Thermal design of the humidification dehumidification desalination system: an experimental investigation,” International Journal of Heat and Mass Transfer, 58:740-748, March 2013. (doi link)

[10] G.P. Narayan, M.H. Sharqawy, S. Lam, S.K. Das, and J.H. Lienhard V, “Bubble Columns for Condensation at High Concentrations of Non-condensable Gas: Heat Transfer Model and Experiments,” AIChE Journal, 59(5):1780–1790, May 2013. (doi link) (preprint)

[11] E.W. Tow and J.H. Lienhard V, “Experiments and Modeling of Bubble Column Dehumidifier Performance,” Intl. J. Thermal Sciences, 80:65-75, June 2014. (doi link) (preprint)

[12] E.W. Tow and J.H. Lienhard V, “Heat transfer to a horizontal cylinder in a shallow bubble column,” Intl. J. Heat Mass Transfer, 79:353-361, Dec. 2014. (doi link) (preprint)

[13] J.H. Lienhard V, “Humidification-dehumidification desalination,” in Desalination: Water from Water, 2nd edition, Chpt.9. Hoboken, NJ: Wiley-Scrivener, 2019. ISBN 978-1-119-40774-4. (preprint)

[14] G.P. Narayan, M.H. Sharqawy, E.K. Summers, J.H. Lienhard V, S.M. Zubair, and M.A. Antar, “The potential of solar-driven humidification-dehumidification desalination for small-scale decentralized water production,” Renewable and Sustainable Energy Reviews, 14(4):1187-1201, May 2010. (doi link) (preprint)